Conformal Mapping & Mobius Transformation
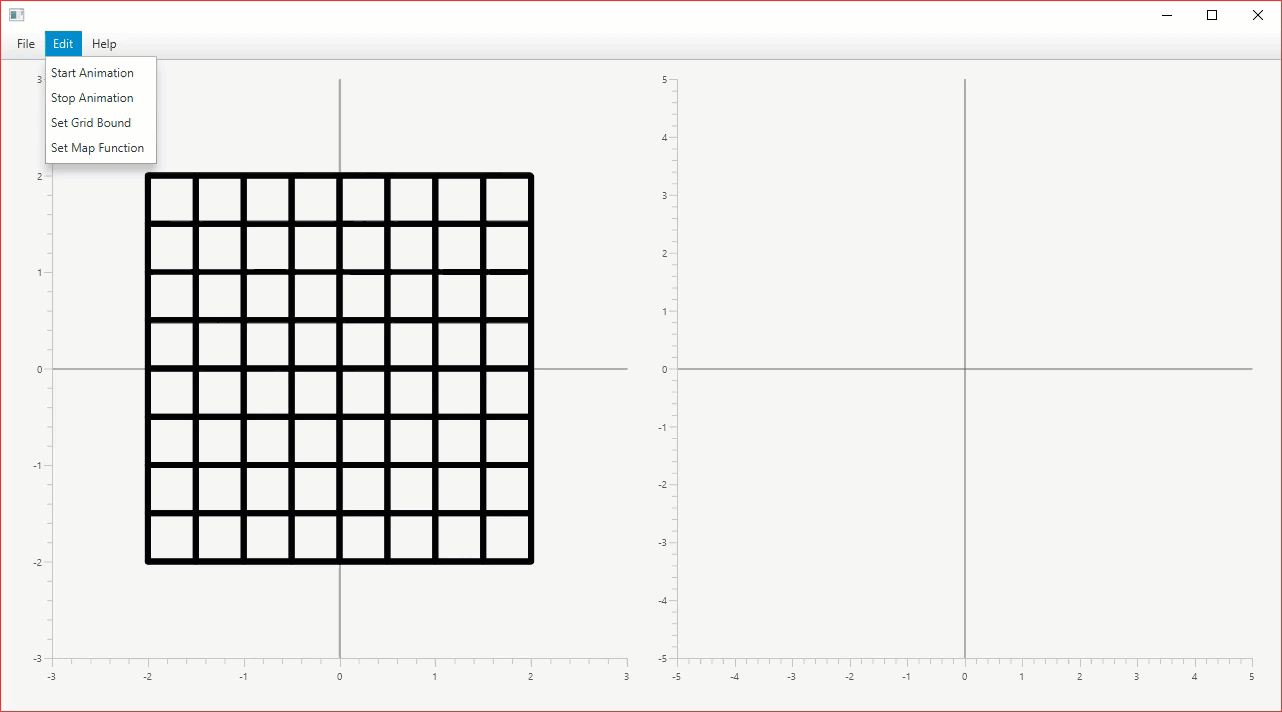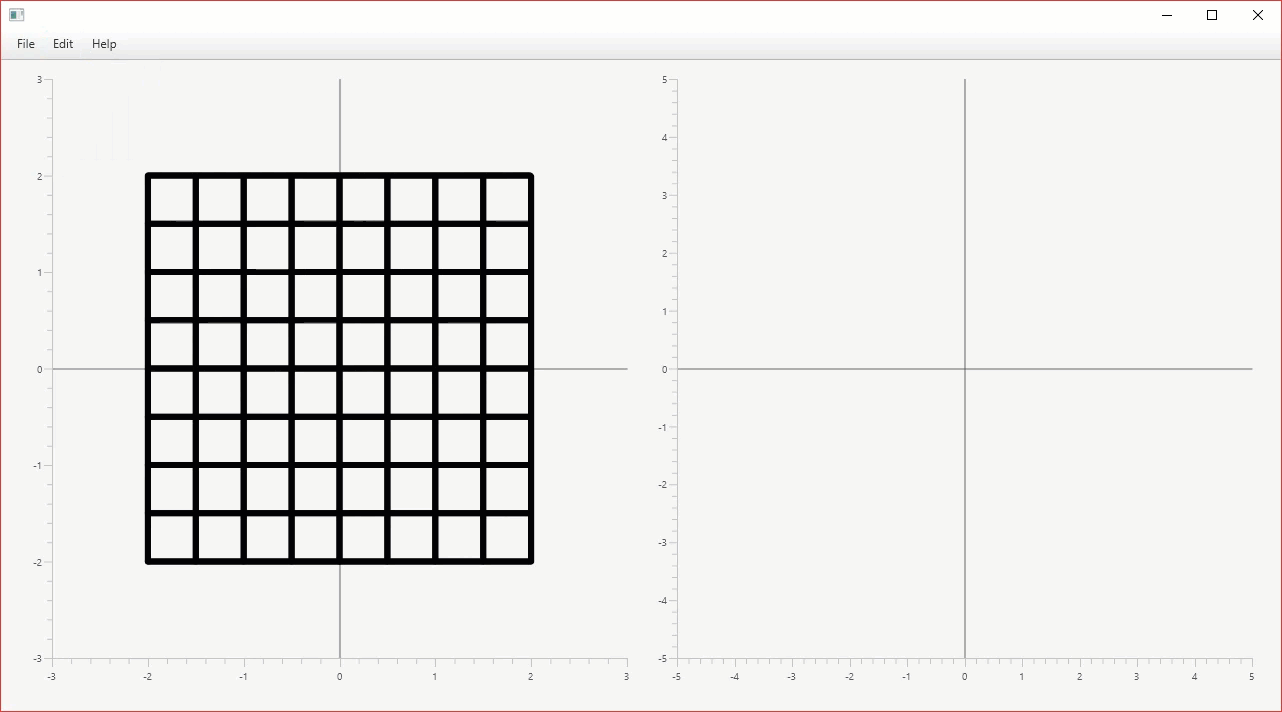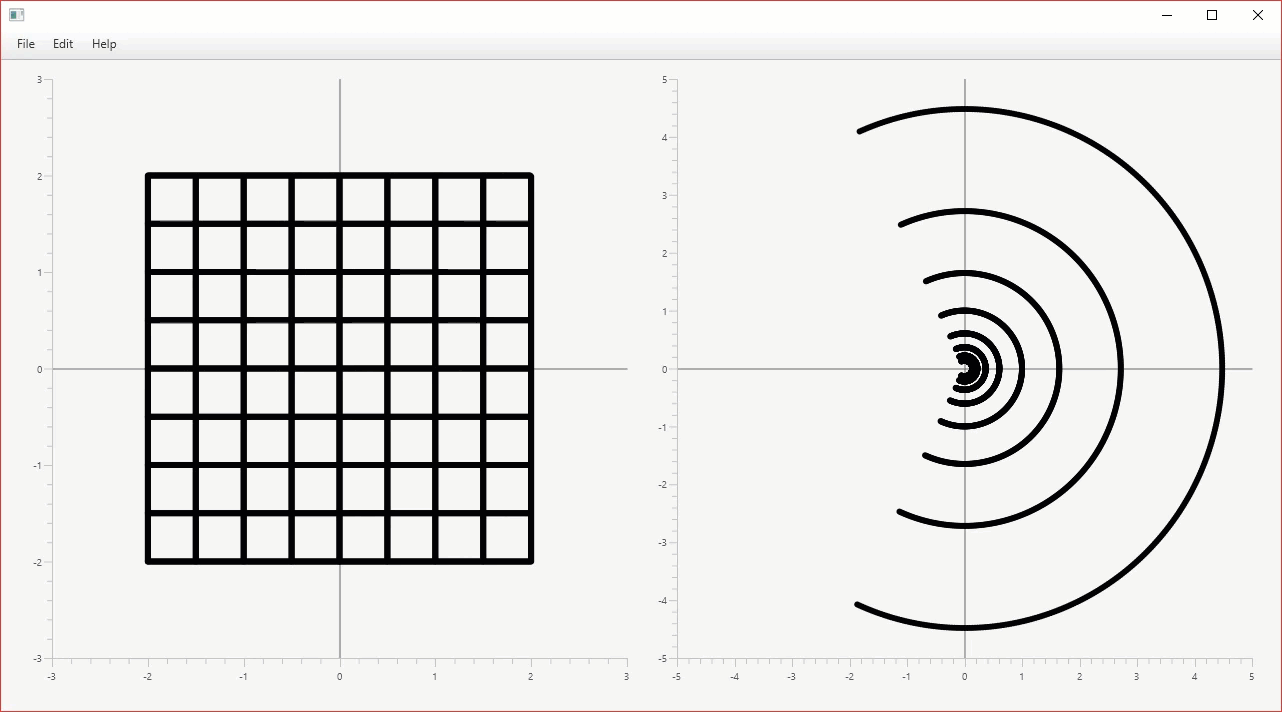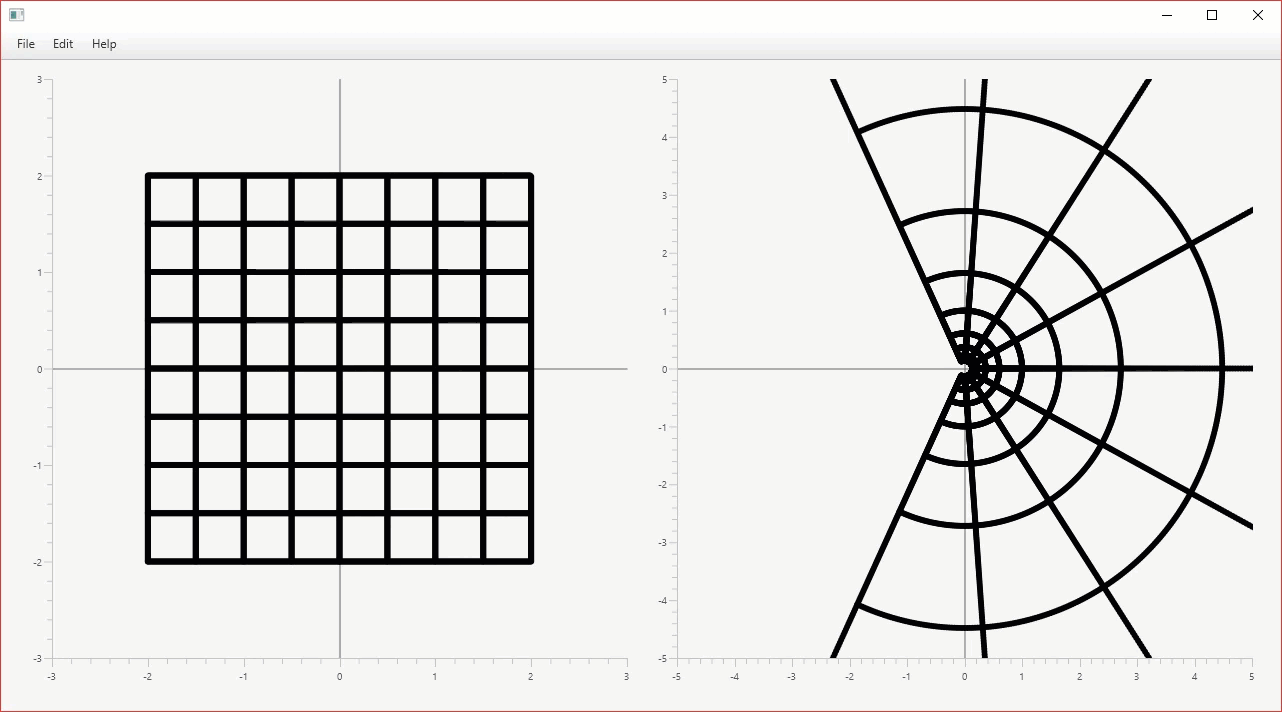
A conformal mapping, also called a conformal map, conformal transformation, angle-preserving transformation, or biholomorphic map, is a transformation w=f(z) that preserves local angles. An analytic function is conformal at any point where it has a nonzero derivative. Conversely, any conformal mapping of a complex variable which has continuous partial derivatives is analytic. Conformal mapping is extremely important in complex analysis, as well as in many areas of physics and engineering.